Gravitational Waves: a New Window on the Universe

Gravitational Waves: a New Window on the Universe

The first ever direct detection of gravitational waves from two black holes [1] was made by the LIGO and Virgo Scientific Collaboration on 14 September 2015.  Measured in units of M_\odot, the mass of our Sun, the two black holes had masses of 36M_\odot and 29M_\odot.  They coalesced to form a single black hole of mass 62M_\odot.  The ‘missing mass’ of {\sim} 3M_\odot was radiated away as gravitational waves.  It was these tiny ripples in space-time that were detected, after their journey of 1.3 billion years on their way to Earth.

Gravitational Waves: a New Window on the Universe figure 1
Figure 1: Plot illustrating the first ever gravitational wave detection, GW150914.  The upper panel illustrates the three phases of the binary black hole system’s evolution.  The middle panel shows the waveform.  The lower panel illustrates the high speeds and small distances involved.

The first detection was announced on 11 February 2016.  It was reported widely in the popular press, with the story given top billing in news outlets the world over.  In the UK, newspaper headlines included ‘Theory of Relativity proved’ (Independent), and ‘So it turns out Einstein was right all along…’ (Guardian).  In this article I will try and put this discovery in its proper context,  explain just why it was so important, and  what the key mathematical ideas were that made it possible.  I will end by looking forward to what might come next.  The connection with space is twofold. Gravitational wave signals themselves are produced by astronomical objects.  But there are also ambitious plans to place a gravitational wave detector in space, as I will describe below.

Figure 1 illustrates what was actually seen.  The images at the very top depict the three stages of the binary’s life: the gradual inspiral, the eventual merger, and the final ‘ring down’ where the final highly distorted back hole settles down into a quiescent state.  The waveforms immediately below show the best-fit template that was used to detect the signal, together with a (very closely matching) waveform produced by a full numerical simulation of the event  (see Section 4 below).  The signal displays the characteristic ‘chirp’ structure expected of a binary coalescence, with the amplitude and frequency gradually increasing up to the final coalescence.

The bottom panel illustrates   just how ‘relativistic’ the event was, both in terms of the high speeds involved, and the very compact nature of the binary in its final stages.  This event is often designated GW150914, according to its date of arrival.  Since then, a second signal has been detected, designated GW151226, again from a binary black hole coalescence event, while a third event, designated LVT151012, was seen in the data, and assigned an 87% probability of being of astrophysical origin [2].

The LIGO detectors are, at the time of writing, continuing to take data, and one can expect that many more detections will follow.

1 History and controversy

This story goes back a long way, all the way to Einstein’s formulation of his theory of general relativity in 1915.  Within just one year, Einstein realised that small disturbances in the space-time would propagate in a wave-like way, at the speed of light [3]. He did this by writing the space-time metric g_{\mu \nu} as a small perturbation h_{\mu \nu} away from the flat-space metric \eta_{\mu \nu} of Minkowski space-time:

(1)   \begin{equation*}g_{\mu \nu} = \eta_{\mu \nu} + h_{\mu \nu} .\end{equation*}

This ansatz was then inserted into the full field equations of general relativity, and the equations linearised in h_{\mu \nu}, to obtain a wave equation for the perturbations.

However, this did not immediately lead to general acceptance of the notion of gravitational waves.  There was a suspicion that these metric perturbations did not actually correspond to anything real.  This suspicion was motivated in part by one of the fundamental features of the theory of general relativity itself – the principle of general covariance. In essence, this says that one can write down the equations of general relativity in any coordinate system one likes, and the equations retain their same (tensorial) form.  Indeed, in order to obtain the wave equation for the metric perturbation in a simple form,  an infinitesimal coordinate transformation is employed, from the coordinate system x^\mu to a new one  x^{\mu '}:

(2)   \begin{equation*}x^\mu \rightarrow x^{\mu '} = x^\mu + \xi^\mu(x^\nu) ,\end{equation*}

with the small quantity \xi^\mu itself satisfying the wave equation.  Given this, it is  easy to see how one might worry that gravitational waves are just a ‘trick of the maths’.  The British astronomer Sir Arthur Eddington once mischievously remarked that gravitational waves ‘travelled at the speed of thought’.

The debate over the reality of gravitational waves was not put to rest until decades later.  The arguments put forward in the 1950s by Pirani and others were influential – see the review by Saulson [4].  Pirani argued that a passing gravitational wave would modulate the (proper) distance between two freely floating test particles.  He noted that the exchange of light signals between the two particles could even be used to measure this modulation, an idea that actually underpins modern gravitational wave detectors.

It eventually became clear to all that gravitational waves are real, and that they carry energy, momentum and angular momentum. For weakly gravitating systems with speeds much less than that of light, there is even a simple formula, first derived by Einstein himself, that gives the gravitational wave luminosity of a body

(3)   \begin{equation*} \dot E = \frac{1}{5} \langle \dddot {{ I} \kern -0.4em\raise 0.25ex \hbox{--}}_{ij} \dddot {{ I} \kern -0.4em\raise 0.25ex \hbox{--}}^{ij} \rangle, \end{equation*}

where  {{ I} \kern -0.4em\raise 0.25ex \hbox{--}}_{ij} is the (trace-reduced) quadrupole moment tensor, with the quadrupole moment tensor itself being given by an integral over the mass density \rho of the source: I_{ij} = \int \rho x_i x_j \,\mathrm{d} V, and the dots denote time derivatives.

Gravitational Waves: a New Window on the Universe figure 2
Figure 2: Gradual shift in the time of periastron (time of closest approach) due to gravitational wave emission, in the Hulse–Taylor binary system. Figure from [6], using data from [7].
The validity of this formula was verified in spectacular style through careful observation of the Hulse–Taylor binary system.  This is a binary star system consisting of two small compact stars known as neutron stars.  One of these neutron stars emits a narrow beam of radio waves, and so is seen as a so-called pulsar, allowing its timing properties, and those of the binary system itself, to be studied in great detail.  Careful observation of its timing has revealed that the binary orbit is shrinking at a rate consistent with the emission of gravitational waves, with the luminosity  given by equation (3); see e.g. [5].

The evolution of the binary orbit  is illustrated in Figure 2, where the gradual shift in time of periastron (the point of closest approach of the two stars) is plotted as a function of time.  The agreement between theory and observation is striking. This provided indirect but powerful observational evidence for the existence of  gravitational waves, and earned Hulse and Taylor the 1993 Nobel Prize in Physics.

2 Sources of gravitational waves

The Hulse–Taylor binary system is now just one of several Galactic binaries where the effects of strong gravity can be probed via pulsar timing [5].  In all these systems, the gravitational waves emitted are much too weak, and at too low a frequency, to be detected by the ground-based detectors like LIGO and Virgo.  However, we can expect similar binary systems, most probably in other galaxies, to have shrunk to the point where the gravitational wave emission is stronger, driving the systems all the way to merger and coalescence.  Exactly the same idea applies to binaries consisting of a pair of black holes, or even a black hole with a neutron star.

Such gravitational wave sources are known as compact binary coalescences in the jargon of the field.  The first two gravitational wave detections (and, so far, the only two) were of precisely this type, both being black hole binaries [1, 8].  But there are other sorts of gravitational wave sources that one can look for, traditionally described as bursts, continuous sources and a stochastic background.

Burst sources are just that: short sharp bursts of gravitational waves, probably produced in some sort of violent explosive event.  A possible source of such signals are supernovae explosions.  These occur when a main-sequence star comes to the end of its life, with its inner core collapsing under the influence of gravity.  The outer layers are blown away, giving rise to a supernova remnant.  Figure 3 shows the Crab nebula, the remains of a supernova explosion that was observed in  1054\mathrm{AD}. Such explosions occur at a rate of around 0.01 to 0.1 events  per year in our galaxy [9].  If the inner core collapses in an asymmetric manner,  gravitational waves will be emitted.   Large-scale numerical modelling has been carried out to try and predict just how strong the gravitational wave emission might be (see e.g. [10]).  The situation is complicated by the fact that the exact mechanism by which a supernova explosion occurs is not well understood.  The successful detection of a gravitational wave signal from such an implosion would help clarify this mechanism.

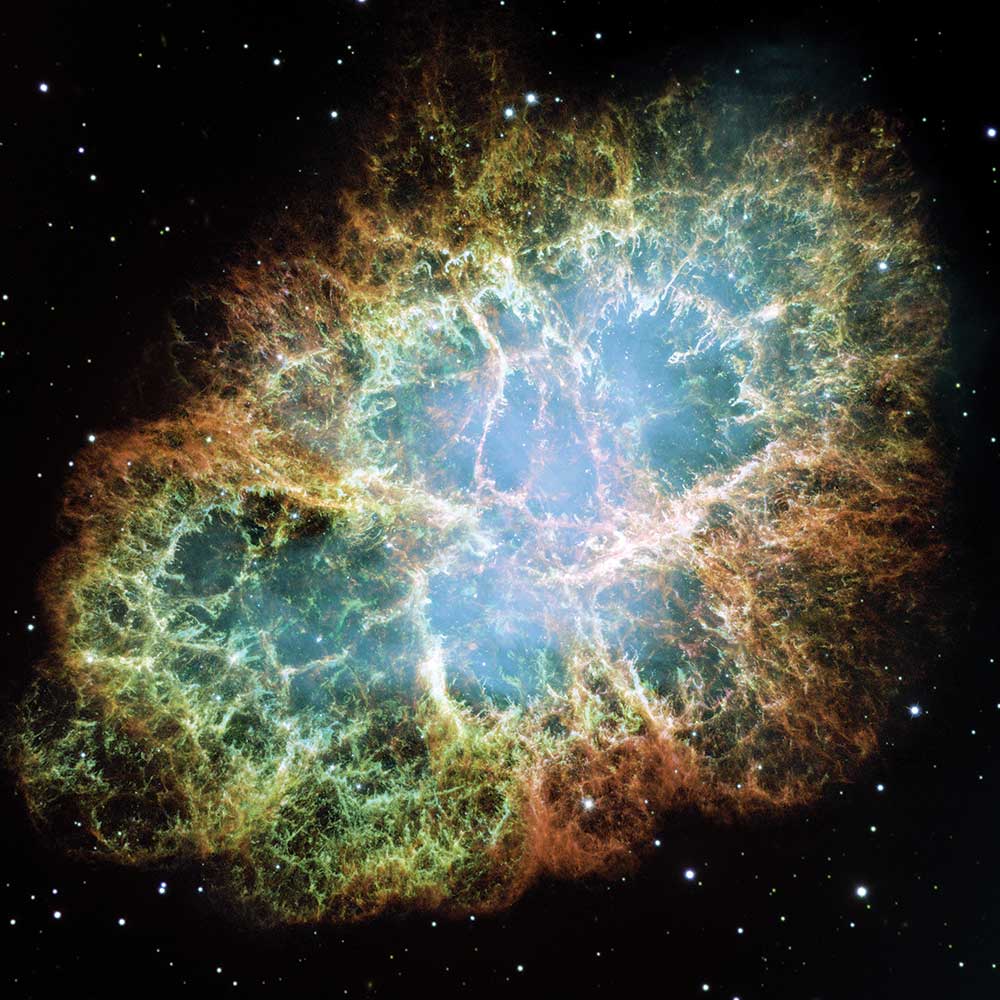
Crab nebula
Figure 3: Image of the Crab nebula, produced in a supernova explosion that was observed in 1054\mathrm{AD}.  Such explosions might produce detectable levels of gravitational radiation.

Continuous sources of gravitational waves are sources that last much longer than the timescales of days to years on which the searches themselves are carried out.  The main candidate for such signals are spinning neutron stars, where the time-variation of the quadrupole moment tensor needed for gravitational wave emission (see equation (3))  is supplied by a non-axisymmetric deformation of the mass distribution.  This may be caused by stresses in the solid/magnetised portions of the star, or else by some small level of excitation of one or more normal modes of oscillation.  Such signals are likely to be weak, but, if detected, will provide information on the state of matter at the very high densities to be found inside neutron stars [11].  There is just such a pulsar at the centre of the Crab nebula of Figure 3, spinning at a rate of about 30 times a second.  Non-detection of gravitational waves from the Crab pulsar has allowed us to show that no more than {\sim} 0.2\% of its total energy budget is going into gravitational waves (the rest is probably radiated electromagnetically); see [12].

Finally, the so-called stochastic background refers to a sea of gravitational radiation where many signals arrive at the detector, overlapping in the frequency and/or time domains, so that individual sources cannot be identified.  Such a background may be of astrophysical origin, e.g. the signal from many weak binary coalescences, or, more interestingly, of primordial origin.  We know of just such an electromagnetic signal: the famous cosmic microwave background, which encodes information on the state of the Universe when it was about 380,000 years old.  The corresponding primordial gravitational wave background potentially encodes information from when the Universe was much younger, less than a second old [13].

3 Gravitational wave detectors

With the realisation that gravitational waves were real and might be able to tell us something interesting about the Universe, came the desire to directly detect them.  The pioneer in this field was Joseph Weber. Starting in the 1960s, Weber built a series of bar detectors.  These were heavy metal cylinders, of length {\sim} 1m, isolated as effectively as possible from seismic noise.  The key idea was that a passing gravitational wave would exert a small but potentially detectable tidal force on the bar, setting it into oscillation. Weber  claimed to have made successful detections, but his findings could not be reproduced by other experimental groups, and were not considered credible.

A limitation of the bar detector technology was size: gravitational effects are tidal, so a bigger detector makes for a stronger signal.  But there are obvious practical limitations as to how one can build bigger and bigger bars.   This consideration was a major driving force behind a switch to an alternative detection technology: %the laser interferometer, for which the L and the I in LIGO stands for (Laser Interferometer Gravitational Wave detector!).  the laser interferometer, which is the L and I in LIGO (Laser Interferometer Gravitational-Wave Observatory).All of the large modern gravitational wave detection experiments  employ this technology.

Gravitational Waves: a New Window on the Universe figure 4
Figure 4: The LIGO detector: (a) Livingston; (b) schematic.

The idea here goes all the way back to Pirani’s original thought experiment: to employ mirrors suspended on wires as the ‘freely floating test particles’, and use a laser beam to measure the distances between them.  Rather than using two mirrors, they use instead two pairs, one forming the x-arm of the interferometer, the other the y-arm. The laser light is then split in half, with one half being  sent up one arm, the other half of the light up the other, and then the beams are  recombined, producing an interference pattern. A passing gravitational wave would then change the relative lengths of the two arms, producing a time-varying interference pattern encoding the gravitational wave signal itself.

The LIGO experiment in fact consists of two such detectors, one near Hanford, Washington State, and one near Livingston, Louisiana.  Figure 4(a) shows the Livingston detector. The arms are 4km long, giving these experiments a factor 4000 advantage over Weber’s original bar detectors.  After having taken data periodically over the interval 2002–2010, the LIGO detectors were upgraded over the period 2010–2015, which led to the detectors starting to operate in their so-called advanced configurations.  Key elements of these advanced LIGO upgrades incorporated advanced technologies previously developed at the UK–German GEO600 detector, together with research led by a consortium of Australian institutions, thus underlining the truly global nature of the gravitational wave research community.

The first detections were made during the very first Advanced LIGO science run, the so-called O1 of September 2015–January 2016.  At the time of writing, O2 is ongoing, and the data is being analysed.

The (dimensionless) metric perturbation of equation (1) has a ready interpretation in terms of the effect of a gravitational wave on a laser interferometer-type detector.  If h denotes the projection of the metric perturbation onto the detector, then the fractional change in length difference between the two arms, each of length L, is given by (\delta L_x - \delta L_y) / L = h.  The strength of the first detected signal, GW150914, was around h \sim 10^{-21}.  This corresponds to a staggeringly small displacement of the test mirrors of around 4 \times 10^{-18}m, or  about one-thousandth of the diameter of a proton.  This underlines the extraordinary technological sophistication of the LIGO detectors, and explains why we had to wait until now, with advanced detector era technology, to make the first detection.

4 Modelling the signal

The main difficulty in gravitational wave detection is the extreme weakness of the signal being sought.   The raw detector output will consist mainly of noise, and one must perform careful data analysis  to identify the signal of interest.  The main technique used in this regard is matched filtering, where the data are correlated with a large set of templates, representing all the possible signals that might arrive, corresponding to, say, all the possible masses of the two stars in a binary system.

This means that it is necessary to construct accurate templates, which match the actual signal, as predicted by general relativity, to better than one cycle over the period of the observation. In the context of binary coalescence, this amounts to solving the two-body problem in relativity.  The two-body problem in Newtonian theory is very simple, with (for point particles at least) the most general orbit consisting of each star following a fixed elliptical trajectory about the system’s centre of mass.

The corresponding problem in general relativity is much more complex.  Fortunately, in recent years, a lot of progress was made in solving the two-body black hole–black hole problem, providing vital input into the recent detection.  Some idea of the issues involved, and the mathematical techniques of relevance, can be gained from the  depiction at the top of Figure 1 of a binary coalescence event.

At early times, when the stars are far apart, the stars are said to be in the ‘inspiral’ regime, as depicted at the top-left of Figure 1.  In this regime there is a small dimensionless number in the problem, v/c, where v is a typical orbital velocity of one or other of the two stars in the binary system, and c is the speed of light.  The existence of this small parameter allows one to use perturbation theory, in this context known as post-Newtonian theory [14].  The key idea is to write the Einstein field equations in the form

(4)   \begin{equation*}\Box h_{\mu \nu} = \frac{16 \pi G }{c^4} \tau_{\mu \nu} \end{equation*}

where \Box is the wave operator (the d’Alembertian), and G is Newton’s gravitational constant.  The quantity  \tau_{\mu \nu} plays the role of an effective energy-momentum tensor, containing the actual energy-momentum tensor T_{\mu \nu}, together with a quantity \Lambda_{\mu \nu} that contains all the terms non-linear in h_{\mu \nu}:

(5)   \begin{equation*}\tau_{\mu \nu} = |g| T_{\mu \nu} + \frac{c^4}{16 \pi G } \Lambda_{\mu \nu} ,\end{equation*}

where |g| is the determinant of the metric.  The equations can then be expanded order by order in powers of v/c, giving expressions for the gravitational wave field, together with the fluxes of energy and angular momentum radiated away from the source.  The leading order term in the energy flux is nothing more than the flux computed long ago by Einstein, as per equation (3), but there are other terms, with interesting interpretations.  For instance, at 1.5 beyond the leading order, there are terms known as ‘tails’, where outgoing gravitational waves are scattered back off the space-time curvature generated by the source; there are also terms due to a coupling between the spin of the component stars and the system’s orbital angular momentum.

These post-Newtonian expressions are useful, and accurate, when the two stars are sufficiently far apart.  As the merger proceeds, and the orbit shrinks due to the loss of orbital energy to gravitational radiation, there comes a point where perturbation theory is no longer appropriate.  This is the ‘merger’ regime, illustrated in the top-middle section of Figure 1. In this regime one must resort to full-blown numerical simulation.   For many years, the field of numerical relativity was beset with stability problems, but rapid progress was made early in the last decade. This progress was due to a combination of factors.  These include clever choices of the formulation of the Einstein equations, including selection of coordinates well suited to numerical evolution, the use of adaptive (i.e.\ time-varying) mesh refinement of the numerical grid, and clever ways of removing the singularities of the black holes from the computation.  These all went hand-in-hand with advances in computing power.  We are now at the point where several groups worldwide have the technology to simulate binary systems of spinning black holes, following the full coalescence where two black holes merge to form one,  extracting the radiation emitted in the process.

The merger itself is not quite the end of the story.  The single back hole formed will, at first, be highly distorted, and will emit gravitational waves at characteristic frequencies as it settles down into its final quiescent state.  This process is known as ring-down, illustrated at the top-right of Figure 1. In this regime a perturbative approach is again applicable.  In this case, one can write the full metric as a perturbation h_{\mu \nu} away from that of the metric g_{\mu \nu}^{(0)} of the final black hole:

(6)   \begin{equation*}g_{\mu \nu} = g_{\mu \nu}^{(0)} + h_{\mu \nu} .\end{equation*}

The final state of the black hole is in fact known in analytic form as the Kerr solution, named after Roy Kerr its discoverer [15].  Perturbations away from the Kerr solution, known as quasi-normal modes, are found to be rapidly damped by gravitational wave emission, and have characteristic frequencies and damping times that encode information on the mass and angular momentum of the black hole.  It is hoped that future, higher signal-to-noise detections than that of GW150914, will allow precision tests of the Kerr solution, particularly if multiple quasi-normal modes can be detected from one and the same black hole.

Of course, we do not know for sure that Einstein’s theory of general relativity is the correct theory of gravity.  However, we can say that the waveforms of the two binary black hole coalescences detected so far, constructed using the techniques described above, are fully consistent with general relativity [16].  It seems that, once again, Einstein’s theory has passed with flying colours.

5 Summary and outlook

Gravitational Waves: a New Window on the Universe figure 5
Figure 5: Gravitational wave detectors. 

The fledgling field of gravitational wave astronomy looks set to grow, as detectors are improved, and new detectors join the international network.  The Virgo detector, near Pisa, Italy (with arm lengths of 3 km) is currently being commissioned into its own advanced configuration, and is expected to join in the data taking soon.  Meanwhile, the Kagra detector is under construction in Gifu-prefecture, Japan, and it too will start taking data in due course.  There is also a plan to build a third LIGO detector in India; see Figure 5.

All of these ground-based detectors suffer from the effects of seismic noise, particularly at lower frequencies.  A way around this is to build a detector in space.  Plans to do just this are well advanced, with the LISA project (Laser Interferometer Space Antenna) having been selected by the European Space Agency as an L3 class mission, as part of the Cosmic Visions programme.  Scheduled for launch in 2034,  LISA will likely consist of three satellites in a heliocentric orbit,  with laser beams travelling back and forth between the spacecraft; see Figure 6.

Schematic LISA triangle
Figure 6: Artist’s impression of the LISA mission, with its three spacecraft forming the vertices of a triangle, acting as corner stations for laser interferometers.

Crucially, being space-borne, it can be made much larger than the ground-based detectors, with arm lengths of the order of  millions of kilometres. This larger size makes it sensitive at lower frequencies than the ground-based detectors, with best sensitivity in the 10^{-4}–10^{-1} Hz range. As such, it will be sensitive to more massive astrophysical sources, including coalescences between the supermassive black holes believed to reside at the centres of most (perhaps all) galaxies, and the inspiral and coalescence of a small black hole into a much larger one.  It offers the prospect of providing further, even higher quality tests of general relativity in the strongest of strong-field regimes.

There is a strong historical resonance in this story, with the first direct detection of gravitational waves having been made one hundred years after Einstein’s 1915 publication of his theory of general relativity, and  the detection being announced 100 years after his 1916 paper on gravitational waves.  But was the story worthy of the attention it received?  Well, in the view of the author at least, the answer must be ‘yes’.   The detection of gravitational waves from a binary black hole coalescence truly was ground-breaking, for two reasons.  Firstly, it represented a new test of Einstein’s theory of general relativity, both in terms of the direct detection of gravitational  waves themselves, and also as a strong-field test of the existence of black holes, with properties just as predicted by the theory.  Secondly, the detection opens up a new window on the Universe, where gravity itself, rather than light, can be used to learn about the heavens.  The era of gravitational wave astronomy has just begun.

Crucial to this success, alongside remarkable technological advances, was decades of theoretical development, that led to the understanding of the nature of black holes, and an accurate calculation of the gravitational signal they emit when they inspiral and coalesce.  But there are, we hope, many other sorts of gravitational wave signal waiting to be discovered, that will continue to provide a challenge in the field of mathematical modelling of strong sources of gravity.

Ian Jones
University of Southampton

References

  1. Abbott, B.P., Abbott, R., Abbott, T.D., et al. (2016) Observation of Gravitational Waves from a Binary Black Hole Merger, Phys. Rev. Lett., vol. 116, no. 6, p. 061102. DOI: 10.1103/PhysRevLett.116.061102.
  2. Abbott, B.P., Abbott, R., Abbott, T.D., et al.  (2016) Binary Black Hole Mergers in the First Advanced LIGO Observing Run, Phys. Rev. X, vol. 6, no. 4, p. 041015.
  3. Einstein, A. (1916) Näherungsweise Integration der Feldgleichungen der Gravitation, Sitzungsberichte der Königlich Preussischen Akademie der Wissenschaften (Berlin), pp. 688–696.
  4. Saulson, P.R. (2011) Josh Goldberg and the physical reality of gravitational waves, Gen. Relativ. Gravitation, vol. 43, p. 3289.
  5. Will, C.M. (2014) The Confrontation between General Relativity and Experiment, Living Rev. Relativ., vol. 17, p. 4.
  6. Hulse–Taylor binary, https://en.wikipedia.org/wiki/Hulse-Taylor_binary (accessed 5 April 2017).
  7. Weisberg, J.M. and Taylor, J.H. (2005) The Relativistic Binary Pulsar B1913+16: Thirty Years of Observations and Analysis in Binary Radio Pulsars, eds  F.A. Rasio and  I.H. Stairs, Astron. Soc. Pac. Conf. Ser., vol. 328, p. 25.
  8. Abbott, B.P., Abbott, R., Abbott, T.D., et al. (2016) GW151226: Observation of Gravitational Waves from a 22-Solar-Mass Binary Black Hole Coalescence, Phys. Rev. Lett., vol. 116, no. 24, p. 241103.
  9. Sathyaprakash, B.S. and Schutz, B.F. (2009) Physics, Astrophysics and Cosmology with Gravitational Waves, Living Rev. Relativ., vol. 12, p. 2.
  10. Ott, C.D., Abdikamalov, E., Mösta, P., et al. (2013) General-relativistic Simulations of Three-dimensional Core-collapse Supernovae, Astrophys. J., vol. 768, p. 115.
  11. Jones, D.I. (2002) Gravitational waves from rotating strained neutron stars, Classical Quantum Gravity, vol. 19, p. 1255.
  12. The LIGO Scientific Collaboration, the Virgo Collaboration, Abbott, B.P., et al. (2017) First search for gravitational waves from known pulsars with Advanced LIGO,  arXiv:1701.07709.
  13. Maggiore, M. (2000) Gravitational wave experiments and early universe cosmology, Phys. Rep., vol. 331, p. 283.
  14. Blanchet, L. (2014) Gravitational Radiation from Post-Newtonian Sources and Inspiralling Compact Binaries, Living Rev. Relativ., vol. 17, p. 2.
  15. Kerr, R.P. (1963) Gravitational Field of a Spinning Mass as an Example of Algebraically Special Metrics, Phys. Rev. Lett., vol. 11, p. 237.
  16. Abbott, B.P., Abbott, R., Abbott, T.D., et al. (2016) Tests of General Relativity with GW150914, Phys. Rev. Lett., vol. 116, no. 22, p. 221101.
Image credit: Figure 1, Observation of Gravitational Waves from a Binary Black Hole Merger / LIGO / CC BY 3.0
Image credit: Figure 2 Hulse–Taylor binary by Inductiveload / Wikimedia Commons / Public domain
Image credit: Crab nebula / NASA, ESA, J. Hester, A. Loll (ASU)
Image credit: Figure 4 / Caltech / MIT / LIGO Laboratory
Image credit: Figure 5 / Caltech / MIT / LIGO Laboratory
Image credit: Artist’s impression LISA misson  © AEI / MM / exozet

Reproduced from Mathematics Today, October 2017

Download the article, Gravitational Waves: a New Window on the Universe (pdf)

Related Posts

Published

© 2024 IMA. All Rights Reserved. Site by Measured Designs.