The opportunity of editing Mathematics Today was one that I seized gratefully. For the past thirty years, I have enjoyed receiving copies of this general interest mathematics publication (formerly IMA Bulletin), so I am delighted to have this chance of contributing to its ongoing success. It had already been published for twenty years before I subscribed and early issues are still well worth reading.
My predecessor, Professor Linton, maintained and improved the quality of Mathematics Today and I echo the vote of thanks that appeared in December’s issue. All members of the Editorial Board generously and voluntarily donate considerable time and effort, as do the many authors of feature articles, reviews and correspondence. Of course, much credit is due to the Editorial Officer, Rebecca Waters, and other professional IMA staff.
There have been some fabulous and prolific contributions over the years, including recent collections such as the Mathematics Matters case studies, Professor Glendinning’s View from the Pennines, Dr Lawrence’s Historical Notes and David Youdan’s Executive Director’s Report. Other talented authors prefer to remain anonymous, including A. Townie (Urban Maths), Your Doctor (A Doctor Writes …) and ZAG (Enigmaths). Amazingly, the last of these has contributed tricky number puzzles continually for the past 25 years! All Mathematics Today features undergo a formal reviewing process, though we invite and consider any contributions that meet the IMA’s objectives.
I first joined the IMA as the fortunate recipient of a year’s free membership when I graduated from Loughborough with a degree in mathematics. After brief spells in business and industry, I conducted postgraduate research in Liverpool and joined the University of Salford, where I have been ever since. My academic career has presented me with a huge variety of interesting work, not least because maths is such a diverse, challenging and engaging subject. Although I might have missed out on other rewarding careers, I have absolutely no regrets about my chosen vocation.
Like smiling and laughing, mathematics can be highly infectious. It is pleasing when students ask penetrating questions during lectures, frustrating when overtime is needed to investigate the problems and ultimately rewarding if solutions can be found. However, such enthusiasm helps to motivate and inspire other students and this must be a good outcome.
When clearing out the attic recently, I stumbled on a chess set and was reminded of an infectious problem that my grandmother posed to me as a child, but which I never managed to solve. Try it and see whether you can succeed…

Take a chessboard and place counters on all the dark squares in the outer rows and columns as shown in Figure 1. The rule is that any counter may jump over and remove any adjacent counter to land on a vacant square, similar to the rights of kings in the game
of draughts. To win the game, you need to remove all but one counter. It is fun to play the game with chocolates, eating them when removed, though this restricts you to one attempt.
Quite independently, I recently came across an interesting article by Roney-Dougal [1], which led me to a paper by Bialostocki [2] relating to the game of solitaire. This is another familiar and stimulating puzzle, a solution of which the same grandmother showed me many years ago.

An English solitaire board has a symmetrical pattern of 33 holes as represented by the squares in Figure 2. Each hole initially contains a peg (or marble) except for the centre hole. Any peg may jump over and remove any adjacent peg to land in a vacant hole and the object of the game is to finish with just one peg in the centre.
Strategies for solution can be difficult to determine analytically, so attempts to solve the problem focus on exhaustive checking with iterative algorithms. In English solitaire, each hole has a binary status and the board has reflective and rotational symmetry, as a result of which there are between and
unique board positions, few of which can arise during play. Given that nearly twenty years have passed since Deep Blue put up a good fight against world chess champion Garry Kasparov, such calculations must be well within the capacity of modern computers. However, Bialostocki solved the fundamental problem elegantly by applying elementary group theory.
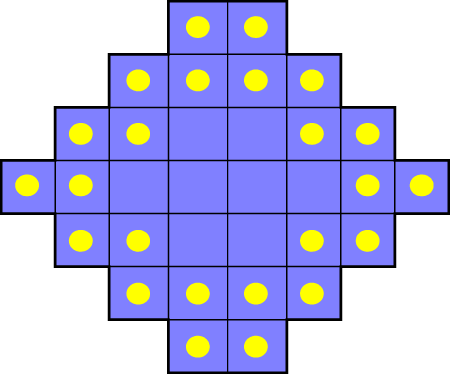
We can use his ideas to solve the draughts puzzle, by noting that the layout in Figure 1 is equivalent to that in Figure 3 after rotating and translating the squares on the chessboard. This now resembles the solitaire puzzle of Figure 2 and the same rules apply for jumping and removing counters. A quick internet search reveals several different solitaire board layouts, including a three-dimensional form that must be fiendishly difficult to visualise.
I could not find the draughts layout of Figure 3 among these, so let us apply Bialostocki’s method. Label the holes using successive diagonals comprising the letters and
, then replace all unoccupied holes with zeroes. The draughts solitaire board thus has the initial layout shown in Figure 4.

Each move transforms the contents of a row or column of three adjacent squares, from two different adjacent letters and a zero to two adjacent zeroes and the third letter, such as . This is equivalent to adding a permutation of
to the contents of these squares, where the set {
} and the operation of addition constitute Klein’s four-group as defined by the Cayley table in Figure 5.
The initial value of this draughts solitaire board is as each element is selfinverse. Moreover,
corresponds to the identity element, so the value of the board is unchanged by all permissible moves such as
. If it were possible to finish with just one peg, the final value of the board would be
or
. As this differs from the initial value of 0, it follows that no solution exists.

I wonder whether Grandma knew about that at the time. It can be surprisingly pleasing to know that no solution exists to a problem, as it helps to focus research in more productive directions. Clearly, I can avoid wasting time trying to solve the draughts puzzle in future, though no doubt I shall be tempted to investigate variants of it next time I clear out the attic. Indeed, as noted by Stewart [3], solutions do exist for the draughts puzzle if either of the corner squares is also unoccupied initially.
A part-time job as a showman in a funfair seems to be beckoning here: perhaps we should add that to the Maths Careers website!
Meanwhile, more important puzzles are challenging us now, some of which may have no solution. Arrow’s impossibility theorem comes to mind with a UK general election looming. Mathematics is playing a leading role in tackling these problems as you will see on the following pages. I hope that you find the contents inspirational and I encourage you to consider submitting articles for future issues of Mathematics Today.
David Percy CMath FIMA CSci
References
- Roney-Dougal, C. (2006) The power of groups, http://plus.maths.org/content/power-groups
- Bialostocki, A. (1998) An application of elementary group theory to central solitaire, The College Mathematics Journal, vol. 29, pp. 208–212.
- Stewart, B. (1941) Solitaire on a checkerboard, The American Mathematical Monthly, vol. 48, pp. 228–233.
Reproduced from Mathematics Today, February 2015
Download the article, Editorial, February 2015 (pdf)



