Westward Ho! Musing on Mathematics and Mechanics

Westward Ho! Musing on Mathematics and Mechanics

Inspired by the village pageants that take place across the county of Cornwall to herald the coming of spring, this issue considers the dynamics of mass synchronous activity. New theories have emerged over the last few decades of how global order can emerge from networks of coupled (near) identical agents. Simulations using such rules can replicate the remarkable observations of swarms of fish or starlings. Yet, a more recent question is how do leaders or influencers emerge within a group of human participants carrying out a synchronous activity. This theory not only has obvious implications in seeking to understand the prescient phenomenon of manipulation of opinion, but also surprising potential application in therapy for extreme psychological conditions.

The small Cornish town of Helston, at the north-western edge of the Lizard Peninsula, is synonymous with its most famous activity, the Furry Dance (or Floral Dance). Held on Flora Day, 8 May each year (unless that falls on a Sunday or Monday, in which case the pageant is moved to the preceding Saturday), the traditional festival involves near continuous dancing from 7.30 in the morning until the early evening. Led by the Helston town band, revellers dance along the streets, through gardens and in out of houses in various costumes at different times of the day. Traditional ‘Helston-born’ dancers wear lilies of the valley; gentlemen on the left, flowers pointing upwards, ladies on the right and upside down. The town is decorated with sycamore branches and, in a special dance at 10.00 am, local children dress in white – the girls wearing flowers in their hair to match their school’s colours.

Helston
Helston

The origins of Flora Day and the Furry Dance are not clear. A play performed in the morning is called the Hal-an-Tow (with the last syllable rhyming with now, rather than toe), the name probably deriving from the Cornish for ‘eve of the fattening time’. It is similar in spirit to a Mummers play as featured in a previous Westward Ho! Important characters include St George, Robin Hood and his Merry men, and the local Saint, Michael. Reference is made in the script to the raid on nearby Newlyn by the Spanish in 1595.

The word Furry itself has no direct meaning in the Cornish language but is thought to have similar origins to ‘feur’ in other Celtic languages which literally means grass, but is also used to signify a festival.

Similar traditions once occurred in the nearby towns of Lizard and Penryn, but have since died out. There also appear to be connections with other May-time festivals in the south-west peninsula, including the ‘Obby ‘Oss festival in Padstow and the Hunting of the Earl of Rone in Combe Martin.

The chief reason for the fame of the Furry Dance is the distinctive tune played throughout the day by the town band. In 1911, a London-based composer, Katie Moss, visited Helston, and joined in the Furry Dance. Inspired, on the train home she wrote words to (a modified version of) the tune in order to describe her experience. She published her composition as ‘The Floral Dance’, an extract from which goes:

As I walked home on a Summer night
When stars in Heav’n were shining bright
Far away from the footlight’s glare
Into the sweet and scented air
Of a quaint old Cornish town

I thought I could hear the curious tone
Of the cornet, clarinet and big trombone
Fiddle, ‘cello, big bass drum
Bassoon, flute and euphonium
Far away, as in a trance
I heard the sound of the Floral Dance

The song soon became a popular classic and today is thought to be the most played tune by brass bands all over the UK. The song had a resurgence in the 1970s, through an arrangement released as a single in 1977 by the Brighouse & Rastrick Band (from the opposite end of England). Picked up by the Radio 2 morning DJ, Terry (later, Sir Terry) Wogan, this instrumental reached the public’s hearts. It would become a massive hit, kept only from the coveted Christmas Number 1 spot by Paul McCartney’s blockbuster ‘Mull of Kintyre’.

According to their webpage, surviving members of the 1977 era band still talk of their experience appearing on Top of the Pops, being enthralled by the burlesque dance group Legs and Co. and a very young Bob Geldof. Led by conductor Derek Broadbent, they were the only artists to perform live on that show. They were straight back on the coach to Yorkshire, with no time for an after party, because they all had proper jobs to go to in the morning. One of the reasons for this unlikely hit was the way that Terry Wogan would sing along live on air, using Katie Moss’s lyrics. In fact, by popular request, Terry released his own sung version in 1978, accompanied this time by the Hanwell Brass Band. But, by then, the public had lost interest and the accompanied version would only reach number 21 in the pop charts.

I digress. Whenever I witness mass synchronous activities, I always wonder how people keep to time. For example, although I am not aware of any particular dance steps associated with the Furry Dance, most traditional English dances involve a repeated sort of shuffling, half skipping step, successively on each foot. When part of a large, unstructured procession, how does one decide whether to place the left or the right foot on a given beat? Presumably by following the person in front.

I also recall the childhood game, in which I often participated on the playground in primary school: follow the leader. Here the head of a small chain of children would weave in whatever direction they saw fit, perhaps lifting a sundry limb or two into the air, perhaps carrying out same strange gait or hand gesture. Each subsequent member of the chain was then obliged to copy as best they could. A wave of human motion would ensue, from front to back, rather like the Mexican wave in sports stadia, where successive sections of the crowd momentarily stand and lift both hands in the air.

murmuration
Figure 1: Starling murmuration at dusk

Following a musical beat is different, though, as this requires synchronous rather than progressive behaviour; all the left feet at once, then all the right feet, and so on. Suppose you were part of the merry throng in Helston on Flora Day, weaving in and out of houses, up and down the streets. You hear the music, but the band is out of sight. How do you decide whether to put your left or right foot down on the next beat? As you look around at the mass of revellers you want to conform, not to be the lone dancer who is (half a period) out of step. So you change step. But what is stopping the dancers next to you, similarly changing step as they notice your foot movements? So how does the apparent synchronisation of an entire crowd happen?

This is a rather trivial example of spontaneous synchronisation, because everyone is being driven by hearing the same music. But there are numerous other examples of synchronisation in technology, nature, and human behaviour in which there is no-one playing the role of the conductor, keeping the beat. Illustrations of this phenomenon include spontaneous rhythmic clapping during rounds of applause, the simultaneous repeated on-off illumination of communities of fire flies and the synchronous firing of neurons within certain brain regions. This theory was brought to public attention some 15 years or so ago with the publication of the book Sync by the great mathematical populariser Steven Strogatz [1]. Strogatz was also among the team of authors who published a Nature paper that claimed the infamous wobbling of London’s Millennium Bridge could be explained by the synchronisation of pedestrian footsteps [2]. (However, more recent theories by my colleague John Macdonald at Bristol University, among others, has cast doubt on synchronisation being the primary cause of that particular instability [3].)

A related phenomenon is the emergent, seemingly coordinated, synchronous spatio-temporal behaviour that can be observed in swarms of birds, fish and insects. One of the most striking examples is the remarkable murmuration of starlings that can be observed at sunset on the Somerset levels, as well as elsewhere at other roosting sites across the UK (see Figure 1). The mathematics of such spatio-temporal patterns will likely form the subject of another Westward Ho! But for this piece, we will restrict attention to the straightforward temporal synchronisation.

The simplest example of temporal synchronisation is the Huygens’ clock phenomenon, between two natural oscillators connected through weak coupling. During the 17th century Huygens, the inventor of the pendulum clock, observed that two such devices either hanging from a beam or sat on a board between two chairs seem invariably to synchronise their phase. The same phenomenon extends to multiple oscillators, as I had the opportunity to witness a few years ago in an afternoon conference excursion to the Great Wall of China. A small group of mathematicians and physicists were taking shelter in the gift shop during a downpour, when we observed a table of identical maneki-neko, or ‘Chinese lucky cats’, set out in a regular array. As shown in Figure 2, we observed how the waving arms of the column of cats in the middle of the table (at the top of the image) were perfectly synchronised. Moreover we could disturb any one of these arms in any way we liked, and eventually it would fall into line with its brothers and sisters. In the figure shown, the gold coloured cat in the middle of the image has a flat battery and is completely still. The other cats in the foreground appear to be only weakly synchronised to each other, and only weakly synchronised to the far column.

To explain the synchronisation of large sets of coupled oscillators, in the 1970s, Yoshiki Kuramoto from Kyoto University proposed a simple model for the dynamics of N weakly coupled near-identical oscillators (see [4] for the first definitive account). Here, instead of modelling the second-order-in-time inertial dynamics of each oscillator, one simply ignores their amplitude and describes the slow variation of the phase \phi_i of each oscillator. The so-called Kuramoto model thus takes the form

(1)   \begin{equation*} \dot{\phi}_i = \omega_i + \frac{k}{N} \sum_{j = 1}^N \sin (\phi_j-\phi_i), \qquad i=1,2,\ldots, N, \end{equation*}

where \omega_i is the natural (uncoupled) frequency of the ith oscillator, k \ll \omega is the coupling strength and a dot represents differentiation with respect to time. This model, and generalisations of it that allow k = k_i to vary with each pairing and to include the effects of temporal noise, has been found to be remarkably successful in explaining the onset of synchronisation among oscillators with initially uncorrelated phases. The interesting case is when the \omega_i are sampled from a relatively narrow range of frequencies \omega \in [\omega^-, \omega^+] and the coupling strength is weak, k \ll \omega. Then, one typically finds a critical value of the coupling strength k_c, such that for k<k_c the oscillators remain independent. But for k>k_c, some of the oscillators start to entrain each other into coherent limit cycles with identical phase and common period 2\pi/\omega_{\text{lc}}, for some average frequency \omega_{\text{lc}}. As the coupling strength increases, this coherent mode entrains more and more oscillators. In the language of dynamical systems, the coupled synchronous motion becomes a stable attractor of the system, and for increased coupling, this attractor both becomes more stable and has an increased basin of attraction.

The ubiquity of the Kuramoto model in explaining synchronisation in physical systems can be understood by thinking about how one might derive such a model in practice. We can think of \phi_i(t) as an angle coordinate that varies around a near circular orbit of the uncoupled ith oscillator viewed in phase space and I_i(t) is the corresponding amplitude. Then, making an (action-angle) reduction into polar coordinates, the underlying dynamics in the angular direction would have the form

    \begin{equation*} \dot{\phi}_i = \omega_i + g(\phi_i,I_i) \end{equation*}

for some function g, that has zero average over one period 2\pi/\omega_i. In fact, using the method of normal forms, one can find a judicious nonlinear co-ordinate transformation and time-reparametrisation to redefine \phi_i and I_i so that g becomes vanishingly small (beyond all algebraic orders).

maneki-neko
Figure 2: Huygens’ cats

Now, suppose we define, \psi = \phi_i-\phi_j, and let \Gamma_{ij} be the \phi_i-component of the coupling force between the ith and jth oscillator. Note that the variable \psi is only defined modulo 2\pi, and so the function \Gamma must also be 2\pi periodic. Hence we can faithfully approximate \Gamma by its Fourier series

    \begin{equation*} \Gamma_{ij} (\psi) \approx a^{(1)}_{ij} \sin(\psi + c^{(1)}_{ij}) + a^{(2)}_{ij} \sin(2\psi + c^{(2)}_{ij}) + a^{(3)}_{ij} \sin(3\psi +c^{(3)}_{ij}) + \ldots, \end{equation*}

for some amplitude and phase constants a_{ij}^{k}\geq 0, c_{ij}^{(k)} \in [0,2\pi). If each orbit is near-circular, it is not hard to argue that this expansion must be dominated by its first term. We are also free to choose the origin of all the phase coordinates \phi_i. If we make the natural physical assumption that the coupling force is zero whenever the oscillators have the same phase, then we are free to choose the phase coordinates such that c^{(1)}_{ij}=0 for all 1\leq i,j \leq N. Finally, if we make the simplifying assumption that the coupling constant a^{(1)}_{ij} is the same for each oscillator (or almost the same), and we set this constant value to be a_1=k/N, then we recover the model (1) to leading order.

Studies of synchronisation using generalisations of the Kuramoto model remain a fertile area of active research, for example, in the case where more terms are included in the Fourier expansion of \Gamma(\psi), or where not all oscillators are coupled, but there is a network, with adjacency matrix A_{ij} that describes which oscillators are coupled to which. Interesting cases also arise in neuroscience where the coupling is not through some smooth function \Gamma but through a discrete collection of temporal spikes. A key question that is often posed in such work is how can one optimally introduce a pacemaker to lead the entire ensemble of oscillators into some desired pattern or behaviour. Or how can one optimally introduce rogue oscillators to desynchronise the group. Both of these ideas have natural application in brain science, and in cardiology, in which synchronicity can be advantageous (coordinated central pattern generation or regular heartbeat) or disadvantageous (epilepsy or fibrillation).

But what has this theory got to do with collective human behaviour ensembles, like during the Furry Dance? A recent EU project, a collaboration between mathematicians at the Universities of Naples, Exeter and Bristol and human movement experts in Montpelier, has considered the question of how synchrony arises in human groups carrying out coordinated tasks. They postulate that coordinated motion can have positive benefits in a number of different situations, such as in sport, music and social exercise. They were particularly interested in how the number and nature of a human’s interactions affects their ability to synchronise.

In one ground-breaking laboratory study [5], seven participants sat facing each other. They were told to perform a simple task of repeatedly moving their finger from side to side in regular motion. The collective motion was captured on film, in two conditions, either with everyone having their eyes closed, or with all having their eyes open. In the closed-eye case, the ‘natural’ frequency of oscillation of each participant could be measured. With eyes open, a number of different interaction topologies were tried, as shown in Figure 3. Each interaction pattern was achieved by where the participants were told to fix their gaze, aided by adjustable goggles that restrict the field of view and by the arrangement of the chairs. The participants were told that the aim was that the whole group should try to synchronise their hand movements, both in frequency and phase. But there was no designated leader.

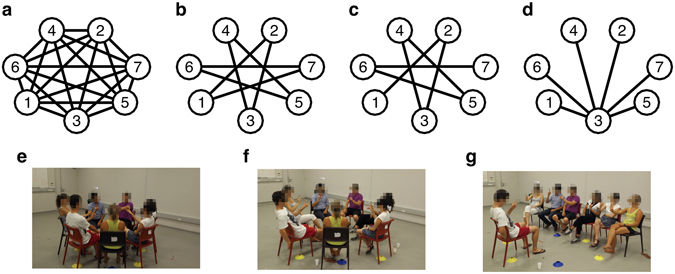
interaction patterns
Figure 3: Experiments in human coordination of hand movements for participants interacting visually in different network topologies (a–d), achieved through arrangement of their chairs (e–g) and instructions on where to fix their gaze. Reproduced from [5], under CCBY license.

It was found that synchronisation was more readily achieved in cases where each participant had a similar natural frequency. It was also found that the topology of the network of lines of sight also greatly affected the propensity to synchronise. Interestingly, the case of all-to-all coupling (Figure 3a) which you might intuitively expect to be more chaotic, was at least as effective as the case where the topology ensures a natural leader (the star graph in d). Also of interest is the fact that the results of these tests could be replicated and explained, using a heterogeneous network of Kuramoto oscillators of the form (1).

Another fascinating question addressed in the same EU project was the extent to which synchronised motion can occur if one or more of the humans were replaced by a computer that is attempting, through automatic control algorithms, to mimic the actions of a human [6]. Although standard feedback control algorithms could be used, the aim was to design a controller that felt human-like, including the kind of perception-cognition pathway delays that a human would display. The solution was to use model-reference control with a (more sophisticated form of) Kuramoto-like oscillator as the reference. It was found that ensembles containing one or more such computer participants (implemented through dots moving on a screen) could be made to perform similarly to entirely human groups.

One possible application of this technology is in rehabilitation robotics. Often physiotherapy following injury involves a patient repeatedly copying the movements of a therapist who is subtly interacting with them, adjusting to their specific impairment. Could a robot be trained to perform the role of the therapist, thus extending the time available for such treatment? A perhaps more surprising motivation for the EU project was in the\linebreak diagnosis and treatment of patients with schizophrenia [7]. It has been documented that schizophrenics have general impairment in interpersonal synchronisation. So the research team, together with clinical collaborators, designed a study in which both healthy and schizophrenic participants interacted one-on-one with a robot. In addition to the above cognition-inspired control algorithm, a humanoid robot was used that was also able to provide social cues, by smiling when synchronisation is almost achieved. It was found that the impaired patients, unlike the healthy ones, had no improved performance in the presence of the social cues. The research suggests possible avenues for both controlled diagnosis and potential treatment of the social deficit present within schizophrenia.

Finally, I would like to ponder on the question of ethics, which is not something we often need to consider within the mathematical sciences. Clearly, any human experiments on campus need full ethical approval, and I have no doubts on both the scientific and societal benefit of the work I have just described on human motion coordination. But I don’t think it takes too much imagination to consider a somewhat less positive application of research on using one or two (secretly) robotic ‘leaders’ to try to influence the behaviour of the masses. While not exactly the same phenomenon, it is much in the news at the moment that certain nefarious organisations may have sought to manipulate the outcome of elections via targeted interventions at certain ‘influential’ nodes in a social interaction network. In the era of sophisticated mathematical models, automated algorithms and big data, perhaps it is time for all of us to consider the possible ethical implications of our research. This is precisely the point argued by Cathy O’Neil in her book Weapons of Math Destruction [8]. It has long been accepted that gaining a deep understanding of ethics is a key part of any degree in medicine. Ethics is also a necessary ingredient of most professionally accredited degrees, not least in engineering. In the age of cyber attacks, ethical education is being introduced to most computer science programmes. But given what is now possible using automatically implemented mathematical models, is it also time that ethics should become a compulsory part of any mathematics degree?

Alan Champneys CMath FIMA
University of Bristol

References

  1. Strogatz, S.H. (2003) Sync: How Order Emerges from Chaos in The Universe, Nature and Daily Life, Hyperion, New York.
  2. Strogatz, S.H., Abrams, D.M., McRobie, A., et al. (2005) Crowd synchrony on the Millennium Bridge, Nature, vol. 438, pp. 43–44.
  3. Macdonald, J.H.G. (2009) Lateral excitation of bridges by balancing pedestrians, Proc. R. Soc. Lond. A, vol. 465, pp. 1055–1073.
  4. Kuramoto, Y. (1984) Chemical Oscillations, Waves, and Turbulence, Springer, Berlin.
  5. Alderisio, F., Fiore, G., Salesse, R.N., et al. (2017) Interaction patterns and individual dynamics shape the way we move in synchrony, Sci. Rep., vol. 7, p. 6846.
  6. Alderisio, F., Lombardi, M., Fiore, G. and di Bernardo, M. (2017) A novel computer-based set-up to study movement coordination in human ensembles, Front. Psychol., vol. 8, art. no. 967.
  7. Cohen, L., Khoramshahi, M., Salesse, N.R., et al. (2017) Influence of facial feedback during a cooperative human-robot task in schizophrenia, Sci. Rep., vol. 7, p. 15023.
  8. O’Neil, C. (2016) Weapons of Math Destruction: How Big Data Increases Inequality and Threatens Democracy, Crown, New York.

Reproduced from Mathematics Today, June 2018

Download the article, Westward Ho! Musing on Mathematics and Mechanics

Image credit: Helston © CountryCollection – Homer Sykes | Alamy Stock Photo
Image credit: Starling Murmuration at Dusk © Thomas Langlands | Dreamstime.com
Image credit: maneki-neko © Alan Champneys
Image credit: Interaction patterns and individual dynamics shape the way we move in synchrony by Alderisio, F., Fiore, G., Salesse, R.N., et al. / Sci. Rep., vol. 7, p. 6846./ CC BY 4.0

Related Posts

Published

© 2024 IMA. All Rights Reserved. Site by Measured Designs.