The game of crosscram was created by Goran Andersson and discussed in an article [1] by Martin Gardner; the game is now more usually referred to as domineering and has attracted the attention of a number of mathematicians since its original presentation in 1974.  An analysis of the various configurations is given in the book Winning Ways for Your Mathematical Plays [2] and the result obtained for best play on boards of different dimensions has been given in an article [3].  However, the mathematical formalism is probably rather difficult for individuals who are not familiar with the notation used in game theory.  Consequently, we adopt, here, a simpler approach, which should enable readers to play interesting games and possibly to explore some of the mathematical ideas behind the sequential placements of the pieces without becoming too enmeshed in the mathematical formalities.  We have used the game successfully in a number of maths masterclass sessions and found that the competitive interactions and strategic aspects are very positive for pupil groups of all ages above ten.

1. The rules of the game

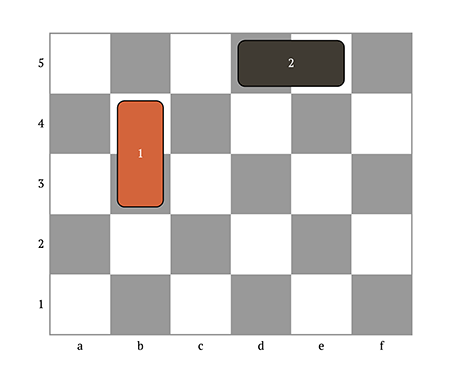
The board consists of a rectangular arrangement of squares (as for chess) but may have any dimension of squares.  There are two players who take alternate turns in placing a domino, occupying two squares, onto the board.  One player (Player A) is restricted to placing her pieces in a vertical orientation and the other player (Player B) is restricted to placing his pieces in a horizontal orientation.  Player A starts the game and each player continues alternately until there is no longer a suitable space for one of the players to place one of their dominoes onto the board.  The player who has made the last legal move wins the game; there cannot be a draw.

A typical game on a or square board lasts less than ten minutes but involves a depth of play that can be engaging, particularly for people who have not played the game before.  However, with best play, the result of the game is already defined by the starting conditions although it is by no means obvious how the sequence of play should evolve, so there are distinct chances for one of the players to reverse the expected outcome.  As a result, the game involves an element of strategic planning and analysis that is both interesting and entertaining but can also be played in a very short time interval.

In this article, we introduce you to some of the fascinating aspects of domineering/crosscram and hope that you will explore some of the possibilities for yourself.  The following sections outline the simple rules and contain some illustrative games and problems.

2. Preliminary considerations

The board size is defined as where is in the horizontal direction, equivalent to the -axis.  If and are equal and both less than , the result of the game is fairly obvious. Player A plays first in all cases and places the dominoes in a vertical position unless there is a special handicap game. It is also convenient to introduce a notation for the squares on the board and this is shown below, using an obvious scheme that can be generalised for any-sized board; it is based on the notation used for a chess board.

The sequence of moves can be easily recorded by writing the symbols for the two squares that have been occupied and examples are given in the next paragraph for some illustrative games on and boards.

3. Illustrative games on the board

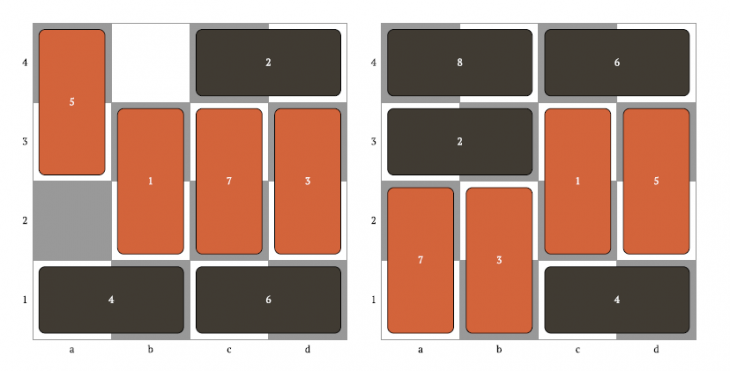
The board provides a convenient introduction to the game and the way of recording the moves, although the actual sequence of play is not particularly optimal in these examples.

Game 1 – b23, 4cd; d23, 1ab; a34, 1cd; c23 and A wins.
Game 2 – c23, 3ab; b12, 1cd; d23, 4cd; a12, 4ab and B wins.

4. The board

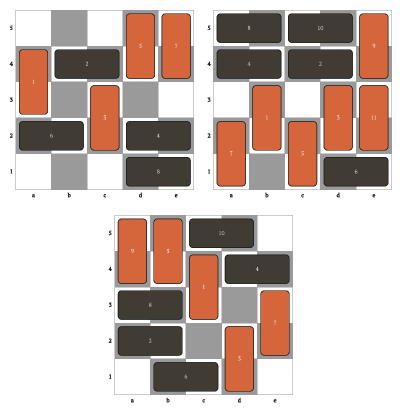
The square board of is the first one to offer an interesting sequence of play with sufficient variability to require strategic considerations.  It is probably best to play a few trial games before reading the rest of this section but we give three illustrative games to indicate the sequence of play.

Game 3 – a34, 4bc; c23, 2de; d45, 2ab; e45, 1de and B wins. Player A has played poorly and Player B has won with three further paired spaces available for him to use!
Game 4 – b23, 4cd; d23, 4ab; c12, 1de; a12, 5ab; e45, 5cd; e23 and A wins.
Game 5 – c34, 2ab; d12, 4de; b45, 1bc; e23, 3ab; a45, 5cd and B wins.

These games are obviously not based on the best play, so the general question is simply stated:

Problem 1:  Who wins with best play on the board?

There are only a few distinguishable opening moves due to symmetry principles but the game tree grows quite rapidly and various strategies can be used to try and create spaces that are not accessible to the other player.  However, it is the final number of dominoes, odd or even, placed on the board that will eventually decide whether Player A or Player B wins the game.

When we have introduced this game in primary maths masterclasses with a group of ‘gifted and talented’ pupils who have not played the game before, the results have been approximately equal for wins by Player A or Player B.  However, with best play either Player A or Player B must always win as it is a game of no chance, similar to various nim-type games; there cannot be a draw, as occurs in the case of best play for noughts and crosses.

5. The board: even more variations

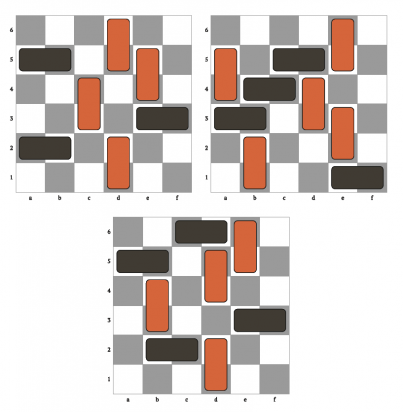
We now consider a   board.  There are clearly many more possibilities for sequential play than for the board and we leave you to explore these possibilities for yourself.  You may also like to solve some of the positions in Problem 2 (Games 6–8)  before tackling the more general question of Problem 3.

Problem 2:  Who should win in each of the following positions?

Game 6 – A: c34, d12, d56, e45 and B: 2ab, 5ab, 3ef with B to play.
Game 7 – A: a45, b12, d34, e23, e56 and B: 3ab, 4bc, 5cd, 1ef with B to play.
Game 8 – A: b34, d12, d45, e56 and B: 5ab, 2bc, 6cd, 3ef with A to play.

Note: from here onwards board references do not refer to sequence of play.

They require some careful thought about the sequence of play, don’t they?  Can you develop a convenient way of analysing the position and deciding on the best play?  The solutions are available at on the Mathematics Today webpage [4].

The general question is, of course:

Problem 3:  Who wins the game on the boards with best play?  Can you explain in simple terms how this conclusion is obtained? Games 9–13 provide unfinished games to help you think about this.

6. Larger boards

The best size for competitive play by inexperienced players is the board, which was discussed in the previous paragraph and takes about ten minutes to complete.  For more serious encounters, the board size can be increased to but the game obviously takes longer.  It will become apparent that certain configurations of available spaces can confer advantages for one or other player and there is a need to keep a close eye on the developing shapes where both players must decide where to protect and where to prevent – it is the judgement of this balance that provides the interest in the sequence of individual plays.

You will not be surprised to learn that there is a systematic way of assessing any position in terms of the shapes of the unfilled squares but a discussion of that procedure is beyond the level of this introductory article.

7. General aspects and interaction strategy

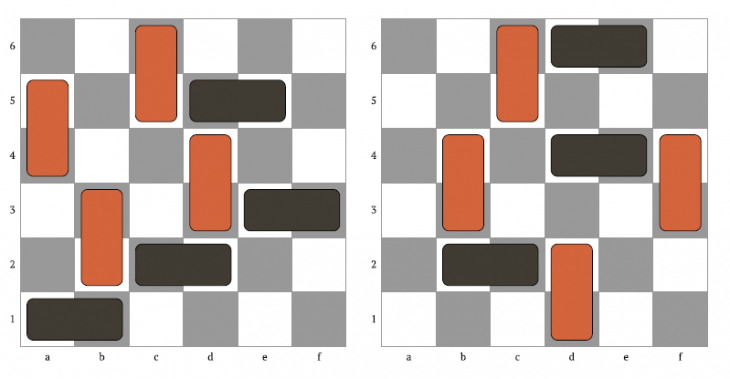
Although the best way to learn the tactical and strategic aspects of the game is to play competitively with someone else, it is not always possible to do this.  We have therefore offered a series of set positions (Games 9 and 10) regarding the sequence of play in the hope that you will be able to absorb some of the analytic thinking in choosing the best moves.  There are no solutions given here but some hints can be found on the Mathematics Today webpage [4].

Game 9 – A: a45, b23, c56, d34 and B: 1ab, 2cd, 5de, 3ef with A to play.
Game 10 – A: b34, c56, d12, f34 and B: 2bc, 4de, 6de with B to play.

8. ‘Advanced’ questions for the reader (no solutions given!)

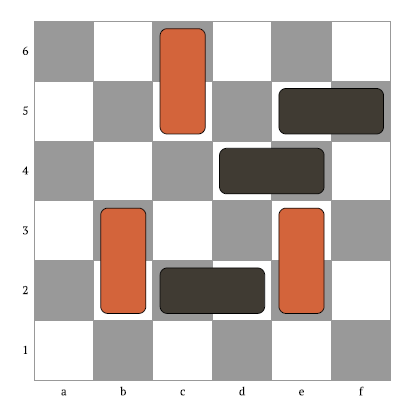
Following on from the last section, it seems appropriate to set a more advanced game using a set position on a board. We provide no hints and leave you to decide the best way to play Game 11.  You can either play with a friend or try to make a complete analysis of the game tree in order to decide who wins with the best possible play.

Game 11 – A:  b23, c56, e23 and B: 2cd, 4de, 5ef with A to play.

Another question involves the start of the game where there are many choices of play.  Games 12 and 13 show two positions at an early stage in the game with only four dominoes played and the remaining area is fully or even multiply-connected.  How do these initial positions affect the subsequent play and who holds the advantage?

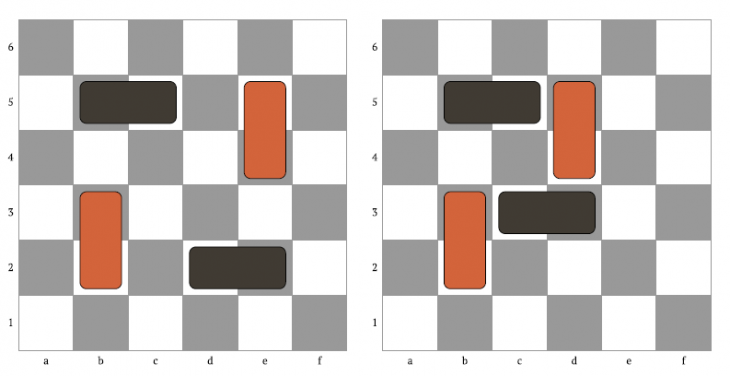
Game 12 – A: b23, e45 and B: 5bc, 2de with A to play.
Game 13 – A: b23, d45 and B: 5bc, 3cd with A to play.

A complete answer to these questions requires a full analysis of all possible games but there are some strategic aspects that arise even at this early stage.  Can you decide who holds the advantage and should win?  We provide no answers but if you are stuck or wish to compare your analysis with our tentative comments, go to the Mathematics Today webpage [4].

9. Summary and possible variants

The foregoing sections have introduced you to a number of features arising in the game of domineering.  These notes should enable you to appreciate the ‘analytic’ elements of the play and possibly to use them in a teaching context or simply to enjoy them as a recreational activity.  If you think that a longer game is preferable, then play with an board and learn to recognise the effective ‘values’ to be associated with different isolated shapes as they are created during the play.  In some respects, this involves concepts that arise in various nim-type games but because it concerns a two-dimensional arrangement there is an increased number of combinations that are `shape-dependent’.  However, a higher-level analysis is possible, so that domineering can be appropriately classified as a game of no chance, such that the result of any position with best play on both sides is already defined.

This article describes the basic game of domineering but it is possible to experiment with alternative rules using the same principles.  For games between players of different expertise it is possible to play with a rectangular board (say ), where the more knowledgeable player is Player A and places her pieces in a vertical orientation; other forms of handicap game with are possible.

An additional game that can be tried is the ‘misere’ version, where the object is to make the opponent place the last piece on the board.  Another unexplored series of variants involves the use of coloured dominoes with rules about placement of adjacent pieces of similar or different colours – there is plenty of scope to experiment with these possibilities.

Domineering may be a less-well-known board game than many others but we hope that this article will lead to a wider interest in its appeal and use in the context of recreational  mathematics.

10. Play domineering

There is a domineering board in this issue of Mathematics Today for you to start playing immediately.

A convenient way to play the game on boards of different dimensions is to visit the website of Jason Davies [5] where there is a general program with an excellent board display and shortcuts to the 13 games mentioned in this article.

John Dore
University of Kent

Chris Woodcock
University of Kent

Illustrations by Jason Davies

References

1. Gardner, M. (1974) Mathematical Games, Sci. Am., vol. 230, p. 106.
2. Berlekamp, E.R., Conway, J.H. and Guy, R.K. (2001) Winning Ways for Your Mathematical Plays, Vol. 1, Chapter 5, A.K.  Peters.
3. Lachmann, M.,  Moore, C. and  Rapaport, I. (2002) Who Wins Domineering on Rectangular Boards, in More Games of No Chance, ed. Nowakowski, R.J., Cambridge University Press,  pp. 307–315.
4. http://tinyurl.com/MTwebpage
5. www.jasondavies.com/domineering/

Reproduced from Mathematics Today, August 2015

Download the article, Domineering:  Comments on a Game of No Chance (pdf)

Published 1st August 2015