Cars are a ubiquitous part of modern life and the UK automotive industry is a key part of the UK economy, producing an average of 1.5 million cars and commercial vehicles every year. With 80% of these products exported overseas, the nation’s car industry racks up an annual turnover of £50 billion.
However, the latest car designs have to keep pace with changes in both technology and legislation. A major area of research and development is reducing the weight of vehicles to lower their carbon emissions and reach government targets. Safety legislation also dictates how the body of the car must behave in a pedestrian collision. One part of a car is particularly subject to both of these constraints: the bonnet. A large, flat panel, it is a prime target for weight reduction and, being at the front of the vehicle, the most likely part for a pedestrian to hit. Yet designs also have to prevent turbulent air created by the car in front causing the bonnet to overly vibrate – an effect known as “bonnet flutter”.
When it comes to designing flutter-free bonnets, that also meet the other criteria, UK car manufacturers, like Jaguar Land Rover, turn to mathematics. New bonnets could be tested on a track, but that would require several versions of the bonnet to be manufactured and put through their paces. By using an area of mathematics called computational fluid dynamics, engineers can test potential designs in a computer, saving both time and money. Mathematical computer modelling is just one part of Jaguar Land Rover’s £1.5 billion annual research and development budget.
Engineers first need to model the air flowing over the car. The hypothetical car sits in a cube containing gridlines – a lattice. The air is treated as a collection of particles able to interact with each other. Particles within the model may represent many actual air particles. Each particle is assigned a value for its speed and its location in space and time. An equation, known as the Boltzmann equation, is then used to give a map of where the particles are likely to be after any given time.
Running these equations through a computer, and stitching these instantaneous maps together, engineers can build up an animation of the likely air flow over the car. Due to the car sitting in a lattice, and the use of the Boltzmann equation, this technique is known as the Lattice Boltzmann Method. The beauty of using a mathematical computer simulation is that engineers can run different scenarios – such as adding a second vehicle – and model the effect on the air flow over the car.
The next step is to calculate the effect of the modelled air flow on the surface of the vehicle. First, the surface is broken up into small 2.5mm cells. The Lattice Boltzmann Method allows engineers to predict how many particles will touch each cell at any given time. This allows them to calculate the likely pressure on each little bit of the car. The engineers now have a highly detailed pressure map over the entire surface of the car and they can see how this pressure varies over time as the dirty air from the car in front flows over it.
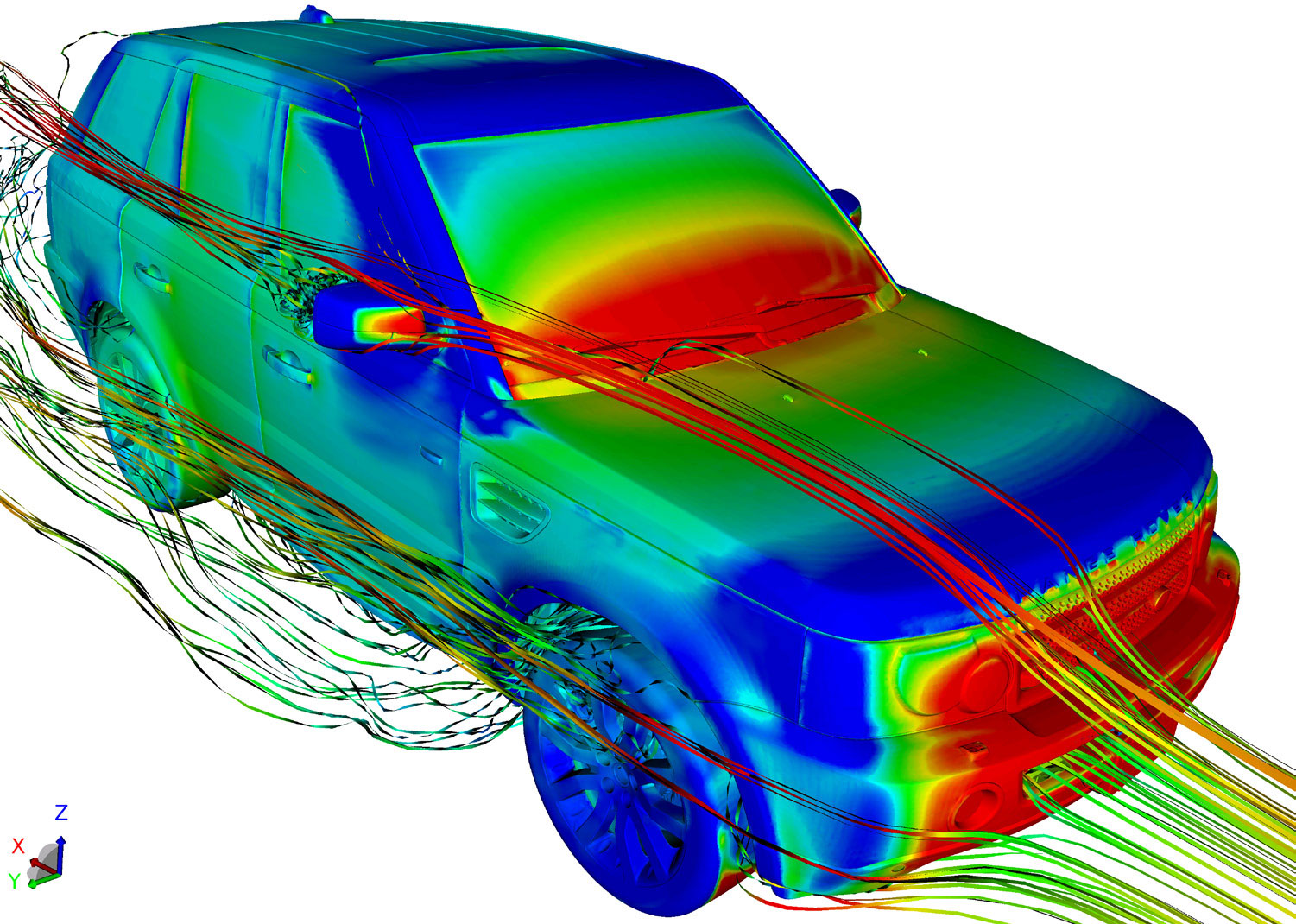When it comes to the bonnet itself, it is modelled using an area of mathematics called Finite Element Analysis. This helps to break the bonnet down into a mesh including elements representing its structure and fixings, such as catches and hinges. The cells in this mesh are larger than the cells in the original pressure map. The mesh is then filled in with data from the original Lattice Boltzmann map to show the pressure on each part of the bonnet.
Variations in pressure on the bonnet will cause it to vibrate and the model can reveal the likely frequency of this vibration. Every system has its own “resonant frequency” – the frequency at which the system vibrates the most. A glass can be shattered if it is exposed to a sustained sound at its own resonant frequency, for example. In the case of a car bonnet, bonnet flutter will be at its greatest if the turbulent air from the car in front causes the bonnet to vibrate at its resonant frequency.
Using these mathematical computer models allows engineers to check if the current design of the bonnet will make it vibrate close to that resonant frequency. If was found to be too close, solutions have traditionally included adding more weight to the bonnet, or making it stiffer, to alter its resonant frequency. However, these changes are in conflict with the carbon emission and road safety targets. Instead, hinges, catches and rubber seals are moved around to increase damping so that the system loses energy faster and can never get up to its resonant frequency. Working virtually, this can be done several times to find the best compromise between cost, performance and regulation.
In this way, mathematics is helping to remove issues from the car design process without the need to physically manufacture and test parts. Mathematics helps engineers get as close to the answer as possible so that any physical changes are small and happen right at the very end of the process, saving time and reducing costs.
Technical Supplement
Boltzmann Lattice Method
The ideal way to model the air flow this situation would be using the Navier-Stokes equations – a set of coupled partial differential equations. These equations work well when describing non-turbulent (laminar) flow corresponding to a low Reynolds number. However, in many real life situations, including automobile design, the flow is not laminar, but turbulent. In the turbulent regime the Navier-Stokes equations are too complicated to currently be solved analytically. Instead, approximations must be made.
One approach is to use the Lattice Boltzmann Method which aims to simulate the dynamics of fluid flow without directly solving the equations. The collection of particles is represented by a particle velocity distribution function and small time steps are implemented to see how that distribution alters. A scattering matrix is used to simulate particle collisions so that the time- average motion of the particles matches what you would expect from Navier-Stokes.
Finite Element Analysis
As the name suggests Finite Element Analysis involves breaking down a shapes into smaller elements, making them easy to work with. For example, a discrete approximation of a circle could be a regular icosagon, consisting of twenty triangles. In theory any shape, including car bonnets, can be described by breaking the structure down into a series of elements. Equations can be used to calculate the physical behaviour of each element. Combining each element at their nodes allows approximate equations to describe the entire shape. There will unknown quantities at the joins between the elements but these can be solved for too. Once you have a description of the overall shape, it can be combined with the map of air pressure. Engineers can then calculate the behaviour of the bonnet, including its vibration frequency.
References
Gaylard, A., Beckett, M., Gargoloff, J., & Duncan, B., CFD-based Modelling of Flow Conditions Capable of Inducing Hood Flutter, SAE Int. J. Passeng. Cars – Mech. Syst. 3(l):675-694, 2010
The IMA would like to thank Adrian Gaylard and the team at Jaguar Land Rover for their help in the preparation of this document.
Printable Version
Download a printable version of this paper here:
